• What and how might you change/revise your model?
• Did you change in your model throughout the modeling process?
• Are there situations where your solution wouldn’t work or your model wouldn’t apply?
• How would you need to change your model to apply to more situations?
• If you had more time, what else would you do?
• Are there any mathematical tools or information that would have been helpful to have?

Standards for Mathematical Practice: Construct viable arguments and critique the reasoning of others.

Edit

Standards for Mathematical Practice:

• Look for and make use of structure.
• Model with mathematics.
• Use appropriate tools strategically.
• Attend to precision.

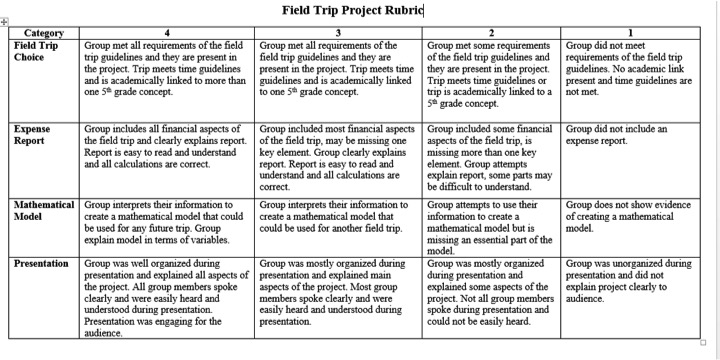
THE LUNCH PROBLEM

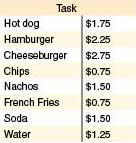You are going to the amusement park. At the amusement park, you will buy lunch. You don’t want to carry coins, only dollar bills, because coins can fall out of your pocket on the rollercoaster. You can purchase any of the items on the menu, but you cannot buy more than one of the same item, and you cannot spend more than $10.00. Using the menu to the left, find out how many different lunches you could buy without receiving any change back.

Doing Mathematics Tasks

• Requires complex and non-algorithmic thinking (i.e., there is not a predictable, well-rehearsed approach or pathway explicitly suggested by the task, task instructions, or a worked-out example). • Requires students to explore and to understand the nature of mathematical concepts, processes, or relationships. • Demands self-monitoring or self-regulation of one’s own cognitive processes. • Requires students to access relevant knowledge and experiences and make appropriate use of them in working through the task. • Requires students to analyze the task and actively examine task constraints that may limit possible solution strategies and solutions. • Requires considerable cognitive effort and may involve some level of anxiety for the student due to the unpredictable nature of the solution process required.

Stein, Mary Kay, Margaret Schwan Smith, Marjorie A. Henningsen, and Edward A. Silver. 2009. Implementing Standards-Based Mathematics Instruction: A Casebook For Professional Development, 2nd ed. New York: Teachers College Press.

http://www.nctm.org/Publications/Teaching-Children-Mathematics/Blog/Tasks,-Questions,-and-Practices/

Standards for Mathematical Practice:

• Look for and make use of structure.
• Model with mathematics.
• Use appropriate tools strategically.
• Attend to precision.

Make assumptions and focus the problem with information and mathematics.

• What information do we already know/need to know to make a model?
• What assumptions do we need to make to build a model?
• What are some important quantities in your situation?
• What mathematical tools could
• you use in your model?

KWI CHART

        Gathering Information

If I knew______, I could figure out_______

(For example, If I knew the cost of a school bus for a field trip, then I could figure out the cost of the transportation.

If I knew how many students and chaperones were coming on the trip, then I could figure out the total number of school buses we would need. )

Standard for Mathematical Practices:Reason abstractly and quantitatively.

 Posing a Mathematical Modeling Problem

Begin with a relatable and interesting Context/Phenomenon that students care about!

Launch: A CLIENT WANTS YOUR CLASS TO PLAN A FIELD TRIP

Brainstorm all the questions you wonder about- Then ask how can we use math to make decisions

Standard for Mathematical Practices: Make sense of problems and persevere in solving them.