Mathematical modeling is a process that uses mathematics to represent, analyze, make predictions or otherwise provide insight into real-world phenomena. Most short definitions we find emphasize this most important aspect, namely the relation between modeling and the world around us. – Using the language of mathematics to quantify real-world phenomena and analyze behaviors. – Using math to explore and develop our understanding of real world problems. – An iterative problem solving process in which mathematics is used to investigate and develop deeper understanding. (Gaimme report, p.8)

Mathematical Modeling can introduce students many ways math can be used to make decisions in our real world and ways to solve problems. See some of the useful models and classroom lessons.

Math Modeling tasks use math to make decisions! Some of the types of modeling problems that we have had success in elementary grades include:
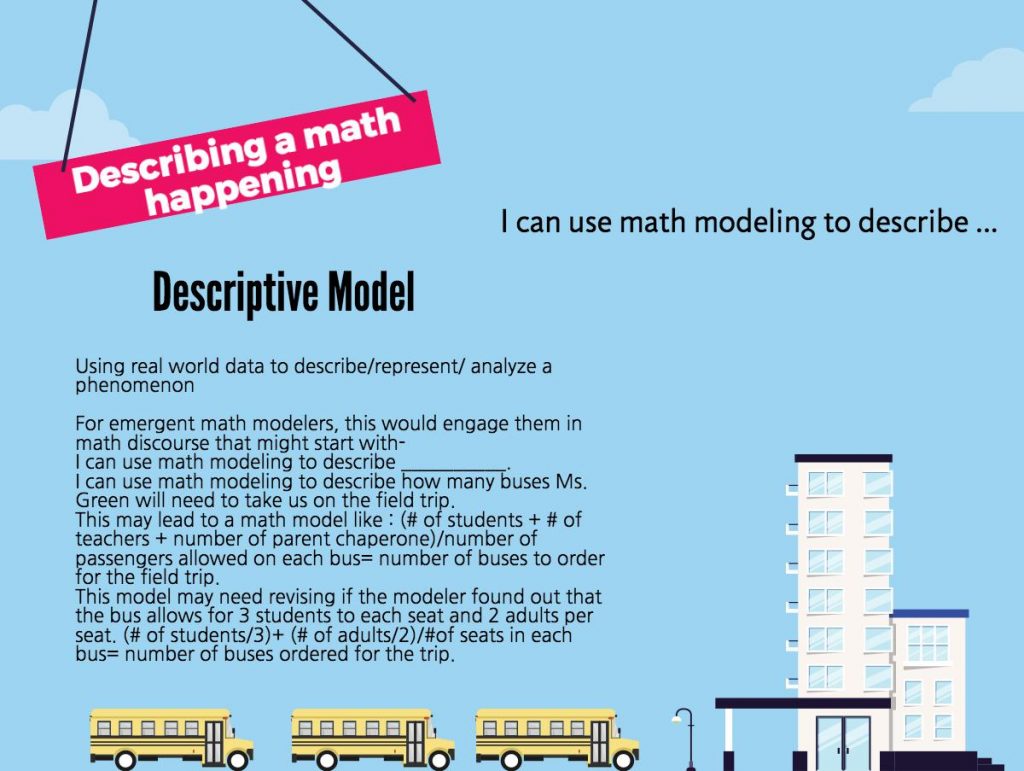
* Descriptive models:  Using real world data to describe/represent/ analyze a phenomenon
For emergent math modelers, this would engage them in math discourse that might start with-
I can use math modeling to describe __________.
I can use math modeling to describe how many buses Ms. Green will need to take us on the field trip.
This may lead to a math model like : (# of students + # of teachers + number of parent chaperone)/number of passengers allowed on each bus= number of buses to order for the field trip.
This model may need revising if the modeler found out that the bus allows for 3 students to each seat and 2 adults per seat. (# of students/3)+ (# of adults/2)/#of seats in each bus= number of buses ordered for the trip.

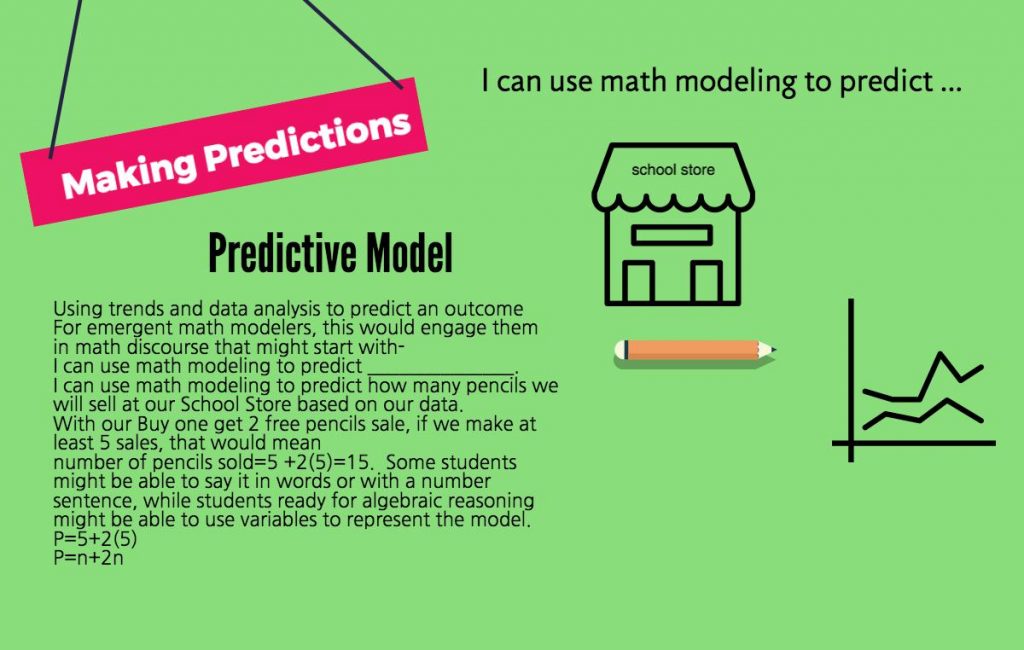
Predictive models: Using trends and data analysis to predict an outcome
For emergent math modelers, this would engage them in math discourse that might start with-
I can use math modeling to predict ______________.
I can use math modeling to predict how many pencils we will sell at our School Store based on our data.
With our Buy one get 2 free pencils sale, if we make at least 5 sales, that would mean
number of pencils sold=5 +2(5)=15.  Some students might be able to say it in words or with a number sentence, while students ready for algebraic reasoning might be able to use variables to represent the model.
P=5+2(5)
P=n+2n

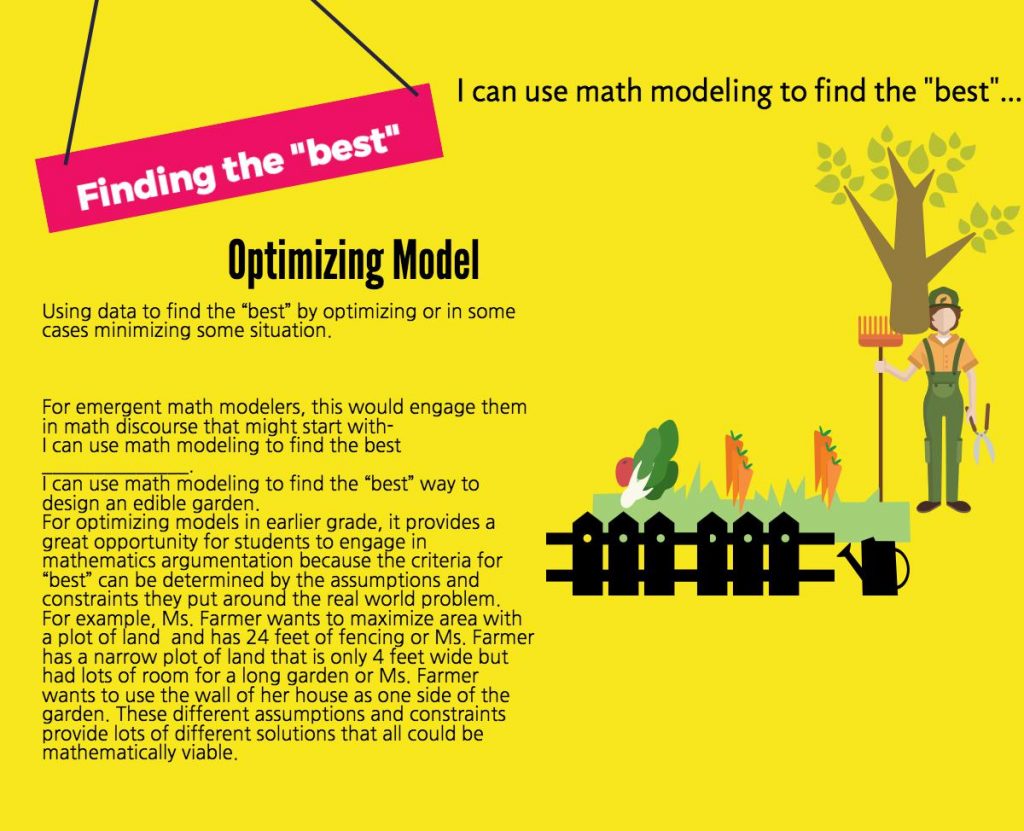
Optimizing models: Using data to find the “best” by optimizing or in some cases minimizing some situation.
For emergent math modelers, this would engage them in math discourse that might start with-
I can use math modeling to find the best ______________.
I can use math modeling to find the “best” way to design an edible garden.
For optimizing models in earlier grade, it provides a great opportunity for students to engage in mathematics argumentation because the criteria for “best” can be determined by the assumptions and constraints they put around the real world problem. For example, Ms. Farmer wants to maximize area with a plot of land  and has 24 feet of fencing or Ms. Farmer has a narrow plot of land that is only 4 feet wide but had lots of room for a long garden or Ms. Farmer wants to use the wall of her house as one side of the garden. These different assumptions and constraints provide lots of different solutions that all could be mathematically viable.

Rating and ranking: Using criteria and mathematical measures as a way to rate and rank options to make decision.
For emergent math modelers, this would engage them in math discourse that might start with-
I can use math modeling to rate and rank to make decisions about  ______________.
I can use math modeling to rate and rank to make decisions about the best college basketball player/team.
I can use math modeling to rate and rank to make decisions about the best vacation spot for our family.
Rating and ranking provides a great way for students to quantify the world around them. This modeling activity is primed for using collected data to make decision. Data scientists use strategies like rating and raking to make important decisions in our daily lives.  Sometimes even exciting decisions like which team to rout for during the March Madnesss game ?

Food for Thought Edible Garden Dream Classroom Planning a Party Touring the City Planning a Field trip Traffic Jam Conserving Energy

 

MM coversation starters

What do you wonder about?
What do you know or notice?
What information do you need?
What assumptions can you make?
How will you solve the problem?Does the solution/model make sense when you go back to your problem? What might you revise and refine in your model to better solve your problem?